Question

At high school, 85% of students are right-handed. Let X - the number of students who are right-handed in a random sample of 10 students from the schools. Which one of the following statements about the mean and standard deviation of the sampling distribution of X is true?A) mean = 8.5; SD = 1.129

B) mean = 8.5; SD = 0.113
C) mean = 85; SD = 1.129
D) mean = 85; SD = 0.113
E) Neither the mean or the standard deviation can be calculated from the information given.

Answer 1

The correct option is (A) mean = 8.5; SD = 1.129.

Explanation:

The random variable X is defined as the number of students who are right-handed.

The proportion of the number of students who are right-handed is, p = 0.85.

The random sample is of size, n = 10.

The random variable X follows a Binomial distribution.

The mean and standard deviation of a binomial distribution can be computed as:

`Mean=np\\SD=√(np(1-p))`

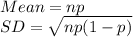
Compute the mean and standard deviation of the sampling distribution of X as follows:

`Mean=n* p=10*0.85=8.5`

`SD=√(np(1-p))=√(10*0.85* (1-0.85)))=√(1.275)=1.12915\approx1.129`

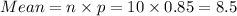
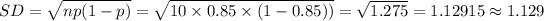
The mean and standard deviation of the sampling distribution of X are 8.5 and 1.129.

The correct option is (A).