Answer:
The variance and standard deviation of X are 0.48 and 0.693 respectively.
The variance and standard deviation of (20 - X) are 0.48 and 0.693 respectively.
Explanation:
The variable X is defined as, X = number of defective items in the sample.
In a sample of 20 items there are 4 defective items.
The probability of selecting a defective item is:
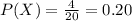
A random sample of n = 3 items are selected at random.
The random variable X follows a Binomial distribution with parameters n = 3 and p = 0.20.
The variance of a Binomial distribution is:
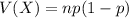
Compute the variance of X as follows:

Compute the standard deviation (σ (X)) as follows:
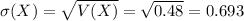
Thus, the variance and standard deviation of X are 0.48 and 0.693 respectively.
Now compute the variance of (20 - X) as follows:
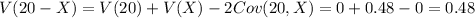
Compute the standard deviation of (20 - X) as follows:
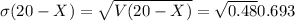
Thus, the variance and standard deviation of (20 - X) are 0.48 and 0.693 respectively.