Question

Each plate of a parallel‑plate capacitor is a square of side 0.0479 m, and the plates are separated by 0.479 × 10 − 3 m. The capacitor is charged and stores 8.11 × 10 − 9 J of energy. Find the electric field strength E inside the capacitor.

Answer 1

Step-by-step explanation:

It is known that the relation between electric field and potential is as follows.

E =
`(V)/(d)`

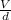
And, formula to calculate the capacitance is as follows.

C =
`(\epsilon_(o) A)/(d)`

=
`(8.854 * 10^(-12) * (0.479 m)^(2))/(0.479 * 10^(-3))`

=
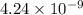`4.24 * 10^(-9)` F

Hence, energy stored in a capacitor is as follows.

W =
`(1)/(2)CV^(2)`

V =
`\sqrt{(2W)/(C)}`

E =
`\sqrt{(2W)/(d^(2)C)}`

=
`(2 * 8.11 * 10^(-9) J)/((0.479 * 10^(-3))^(2) * 4.24 * 10^(-9))`

=
`16.687 * 10^(3) N/C`

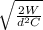
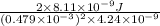
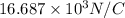
Thus, we can conclude that electric field strength E inside the capacitor is
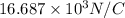`16.687 * 10^(3) N/C`.