Question

. A stone is thrown with an initial speed of 15 m/s at an angle of 53° above the horizontal from the top of a 35 m building. What is the speed of the rock as it hits the ground?

Answer 1

29.04m/s

Step-by-step explanation:

Let the initial speed be u;

Since the motion of the stone is two-dimensional;

Let the horizontal component of the initial speed by
`u_(X)` = u cos θ

Let the vertical component of the initial speed by
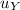`u_(Y)` = u sin θ

Where;

θ = the angle of projection above the horizontal.

Now using one of the equations of motion as follows, let us calculate the maximum height reached (from the top of the building) by the stone which is in the vertical component;

v² = u² + 2as -----------------(i)

Where;

v = vertical speed of the stone at maximum height = 0 [at maximum height, velocity is zero]

u = initial vertical speed of the stone =
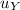`u_(Y)` = u sin θ

a = acceleration of the stone in the vertical direction = -g [ -ve sign shows that is upwards against acceleration due to gravity]

s = h = maximum height reached from the top of the building.

Therefore, equation (i) becomes;

0 = (
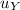`u_(Y)`)² + 2(-g)h

0 = (u sin θ)² - 2gh

=> 2gh = (u sin θ)²

=> h = (u sin θ)² / (2g) -----------------(ii)

From the question;

θ = 53°

u = 15m/s

Take g = 10m/s² and substitute other values into equation (ii) as follows;

h = (15 sin 53°)² / (2 x 10)

h = (15 x 0.7986)² / 20

h = 11.979² / 20

h = 143.496 / 20

h = 7.17m

Therefore, the maximum height reached from the top of the building is 7.17m.

However, the maximum height (H) reached from the bottom of the building (ground) is the sum of the height of the building (35m) and the further height reached (7.17m).

H = 35 + 7.17

H = 42.17m

Now, from the maximum height(H) let's calculate the speed of the rock as it hits the ground using equation (i) as follows;

v² = u² + 2as -------------------(ii)

Where;

v = speed of the stone as it hits the ground

u = initial velocity of the stone from maximum height = 0 [since velocity is 0 at maximum height]

a = acceleration due to gravity = +g [since the rock now moves downwards in the direction of gravity]

s = H = maximum height reached by the rock from the ground = 42.17m

Substitute these values into equation (ii) to get the speed (v) of the rock as it hits the ground;

v² = 0² + 2g(42.17) [Take g = 10m/s²]

v² = 0 + 2(10 x 42.17)

v² = 843.4

v =
`√(843.4)`

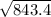
v = 29.04m/s

Therefore, the speed of the rock as it hits the ground is 29.04m/s

Answer 2

30.2 m/s

Step-by-step explanation:

Let ground be the 0 reference for potential energy,and gravitational acceleration g = 9.81 m/s2. Before the throw, the total mechanical energy (kinetic and potential) of the stone is:

`E = E_p + E_k = mgh + mv^2/2 = m(gh + v^2/2) = m(9.81 * 35 + 15^2/2) = 455.85m`

where m is the mass of the stone

According to the law of energy conservation, the total mechanical energy must be conserved. At ground 0, potential energy must be 0. So all of the initial energy is converted to kinetic energy

`E_K = mV^2/2 = 455.85 J`

`V^2 = 2*455.85 = 911.7`

`V = √(911.7) = 30.2 m/s`