Question

Two point charges q and 4q are at x = 0 and x = L, respectively, and free to move. A third charge isplaced so that the entire three-charge system is in static equilibrium. What are the magnitude, sign, andx-coordinate of the third charge?

Answer 1

The answers to the question are

Magnitude = 4/9·q

Sign = Opposite in sign to those of q and 4·q, that is, -ve where q and 4·q are +ve

x coordinate L/3

or
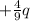`+(4)/(9) q` at x coordinate =
`(L)/(3)`

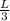
Explanation:

To solve the question, we note that

Force between charges is given by

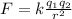`F =k(q_1q_2)/(r^2)`, therefore the force between the two charges q and 4q is

`F = k(q(4q))/((L^2)) = k(4q^2)/(L^2)`

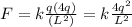
For equilibrium, the charge on the third charge p, will be opposite to those of q and 4·q and the location will be between 0 and L

Therefore the force between the p and q placed at a distance d from q =
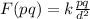`F(pq) = k(pq)/(d^2)` and the force between p and 4q =
`F(4qp) = k(4pq)/((L-d)^2)`

For equilibrium, these two forces should be equal, therefore

`k(qp)/(d^2)`=
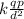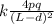`k(4pq)/((L-d)^2)` which gives
`(qp)/(d^2) = (4pq)/((L-d)^2)` and by cross multiplying, we have

(L-d)²× p×q = d²× 4×p×q → (L-d)² = d²× 4 = (L-d)² - d²× 4 = 0 or

L² - 2·d·L -3·d² = 0, which could be factored as

(L+d)×(L-3·d) = 0 Which gives either L = -d or L = 3·d

Since L > d as d is in between 0 and L, then the correct solution is L = 3·d

Since the system is in equilibrium then
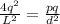`(4q^2)/(L^2) = (pq)/(d^2)` or
`(4q^2)/((3d)^2) = (pq)/(d^2)`

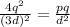
Cancelling like terms gives
`\frac{4}9 q= p`

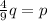
Therefore the magnitude of p = 4/9·q

The location of p is L/3 from the charge q