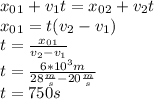Question

two cars are moving at constant speeds in a straight line along a major highway. The first is travelling at 20ms^-1 and the second at 28m^-1. If the second car is 6km behind the first car, how much time will it need to catch up with the first car

Answer 1

`t=750s`

Step-by-step explanation:

The two cars are under an uniform linear motion. So, the distance traveled by them is given by:

`\Delta x=vt\\x_f-x_0=vt\\x_f=x_0+vt`

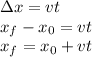
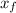`x_f` is the same for both cars when the second one catches up with the first. If we take as reference point the initial position of the second car, we have:

`x_0_1=6km\\x_0_2=0`

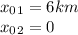
We have
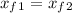`x_f_1=x_f_2`. Thus, solving for t:

`x_0_1+v_1t=x_0_2+v_2t\\x_0_1=t(v_2-v_1)\\t=(x_0_1)/(v_2-v_1)\\t=(6*10^3m)/(28(m)/(s)-20(m)/(s))\\t=750s`