Question

The midpoints of a regular hexagon are connected to form a smaller hexagon. The small hexagon has perimeter $2\sqrt{3}.$ What is the perimeter of the large hexagon

Answer 1

4

Step-by-step explanation:

we can set up a 120,30,30 isosceles triangle than divide it into two 30 60 90 triangles then 2 rt 3 divide it by 12 (the hexagon and the two triangle) then we get 1/6 rt 3 after that we get 1/3 as the side length but since we divide by 2 we need to multiply by two thus getting us 2/3 but to get the perimeter, we need to multiply by 6 and our final answer is 4

Answer 2

The perimeter of the large hexagon is equal to 12 units.

Step-by-step explanation:

Given,

The perimeter of small hexagon =
`2√(3)`

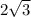
To find, the perimeter of the large hexagon = ?

We know that,

The perimeter of the large hexagon

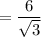`= (6)/(√(3))` × The perimeter of small hexagon

=
`(6)/(√(3)) * 2√(3)`

= 6 × 2

= 12 units

∴ The perimeter of the large hexagon = 12 units

Thus, the perimeter of the large hexagon is equal to 12 units.