Question

A publishing company prints newspapers and magazines. Let NNN represent the number of newspapers and MMM represent the number of magazines the company can print with its daily supply of ink cartridges. 0.5N+2.5M \leq 60000.5N+2.5M≤60000, point, 5, N, plus, 2, point, 5, M, is less than or equal to, 6000 The company wants to print 800080008000 newspapers daily. How many magazines at most can the company print daily with the remaining number of ink cartridges?

Answer 1

800 at most

Explanation:

Answer 2

At most 800 magazines the company can print daily with the remaining number of ink cartridges.

Explanation:

We are given the following in the question:

`0.5N+2.5M \leq 6000`

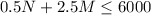
The above inequality gives the relation for daily supply of ink cartridges where N is the number of newspaper and M is the number of magazines.

Number of newspaper to be printed daily,N = 8000

We have to find the number of magazines at most can the company print daily with the remaining number of ink cartridges.

Puting the value in the given inequality,

`0.5(8000)+2.5M \leq 6000\\\Rightarrow 4000+2.5M \leq 6000\\\Rightarrow 2.5M \leq 2000\\\\\Rightarrow M \leq (2000)/(2.5)\\\\\Rightarrow M \leq 800`

Thus, at most 800 magazines the company can print daily with the remaining number of ink cartridges.