Answer: The boiling point elevation of salt water solution is 0.55°C
Step-by-step explanation:
To calculate the mass of water, we use the equation:

Density of water = 1 g/mL
Volume of water = 122 mL
Putting values in above equation, we get:

To calculate the elevation in boiling point, we use the equation:

Or,
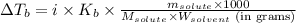
where,
 = ?
= ?
i = Vant hoff factor = 2 (For NaCl)
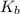 = molal boiling point elevation constant = 0.52°C/m.g
= molal boiling point elevation constant = 0.52°C/m.g
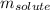 = Given mass of solute (NaCl) = 3.80 g
= Given mass of solute (NaCl) = 3.80 g
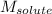 = Molar mass of solute (NaCl) = 58.55 g/mol
= Molar mass of solute (NaCl) = 58.55 g/mol
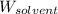 = Mass of solvent (water) = 122 g
= Mass of solvent (water) = 122 g
Putting values in above equation, we get:
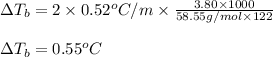
Hence, the boiling point elevation of salt water solution is 0.55°C