Question

Consider the reaction data. A ⟶ products T ( K ) k ( s − 1 ) 225 0.385 525 0.635 What two points should be plotted to graphically determine the activation energy of this reaction? To avoid rounding errors, use at least three significant figures in all values. x 1 = y 1 = x 2 = y 2 = Determine the rise, run, and slope of the line formed by these points. rise: run: slope: What is the activation energy of this reaction? E a = J / mol

Answer 1

Plot ln K vs 1/T

(a) -0.5004; (b) 0.002 539 K⁻¹; (c) -197.1 K⁻¹; (d) 1.64 kJ/mol

Step-by-step explanation:

This is an example of the Arrhenius equation:

`k = Ae^{-E_(a)/RT}\\\text{Take the ln of each side}\\\ln k = \ln A - (E_(a))/(RT)\\\\\text{We can rearrange this to give}\\\ln k = - (E_(a))/(R)(1)/(T) + \ln A\\\\y = mx + b`

Thus, if we plot ln k vs 1/T, we should get a straight line with slope = -Eₐ/R and a y-intercept = lnA

Data:

`\begin{array}{cccc}\textbf{k/s}\mathbf{^(-1)} &\mathbf{\ln k} & \textbf{T/K} & \mathbf{1/T(K^(-1))}\\0.285 & -0.9545 & 225 &0.004444\\0.635 & -0.4541 & 525 & 0.001905\\\end{array}`

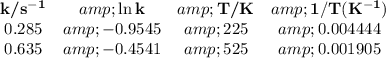
Calculations:

(a) Rise

Δy = y₂ - y₁ = -0.9545 - (-0.4541) = -0.9545 + 0.4541 = -0.5004

(b) Run

Δx = x₂ - x₁ = 0.004 444 - 0.001 905 = 0.002 539 K⁻¹

(c) Slope

Δy/Δx = -0.5004/0.002 539 K⁻¹ = -197.1 K⁻¹

(d) Activation energy

Slope = -Eₐ/R

Eₐ = -R × slope = -8.314 J·K⁻¹mol⁻¹ × (-197.1 K⁻¹) = 1638 J/mol = 1.64 kJ/mol