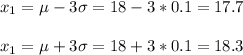Question

Your manufacturing company sells metal nuts and bolts that come together in a package. The diameter of the bolts coming off the factory line follows a normal distribution with mean 18mm and standard deviation 0.1mm. The diameters of the holes in the nuts follow a normal distribution with mean 18.7mm and standard deviation of 0.08mm. Assume that in order for a nut and bolt to fit together the diameter of the hole in the nut has to be between .5 and .9 mm larger than the bolt. Answer all questions to 3 decimal places. You have 5 attempts on numeric answers and 1 attempt on multiple choice answers. 1. Find the z-score for a Bolt of 18.12mm Nut of 18.532mm 2. How many standard deviations away from the average is a bolt with a diameter of 18.08mm? 3. Which is more likely Bolt smaller than 17.84mm Bolt larger than 18.12mm 4. Which is more likely Bolt larger than 18.23mm Nut smaller than 18.548mm 5. About 50% of nuts are smaller than mm For the following questions enter the smaller number first 6. About 95% of bolts are approximately between mm and mm 7. About 99.7 % of nuts are approximatelly between mm and mm

Answer 1

1. z_bolt = 1.2

z_nut=-2.1

2. A bolt with a diameter of 18.08 mm is less than one SD of the mean.

3. It is more likely a bolt larger than 18.12 mm.

4. The bolt larger than 18.23 mm is more likely.

5. About 50% of nuts are smaller than 18 mm

6. About 95% of bolts are approximately between 17.804 mm and 18.196 mm

7. About 99.7 % of nuts are approximatelly between 17.7 mm and 18.3 mm

Explanation:

Bolts: Mean: 18.00 mm SD: 0.10 mm

Holes: Mean: 18.70 mm SD:0.08 mm

1. Find the z-score for a Bolt of 18.12mm Nut of 18.532mm

Bolt

`z=(x-\mu)/(\sigma)=(18.12-18.00)/(0.10)= (0.12)/(0.10) =1.2`

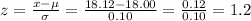
Hole

`z=(x-\mu)/(\sigma)=(18.532-18.70)/(0.08)= (-0.168)/(0.08) =-2.1`

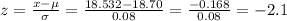
2. How many standard deviations away from the average is a bolt with a diameter of 18.08mm?

Because the SD is 0.1 mm, we can say that a bolt with a diameter of 18.08 mm is less than one SD of the mean (<18.10 mm).

3. Which is more likely Bolt smaller than 17.84mm Bolt larger than 18.12mm

We have to calculate the probability of both.

Bolt #1

`z=(x-\mu)/(\sigma)=(17.84-18.00)/(0.10)= (-0.16)/(0.10) =-1.6\\\\P(z<-1.6)=0.0548`

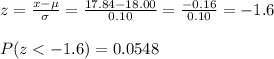
Bolt #2

`z=(x-\mu)/(\sigma)=(18.12-18.00)/(0.10)= (0.12)/(0.10) =1.2\\\\P(z>1.2)=0.11507`

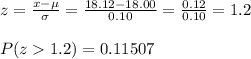
It is more likely a bolt larger than 18.12 mm.

4. Which is more likely Bolt larger than 18.23mm Nut smaller than 18.548mm

Bolt

`z=(x-\mu)/(\sigma)=(18.23-18.00)/(0.10)= (0.23)/(0.10) =2.3\\\\P(z>2.3)=0.01072`

Nut

`z=(x-\mu)/(\sigma)=(18.548-18.70)/(0.08)= (-0.152)/(0.08) =-1.9\\\\P(z<-1.9)=0.02872`

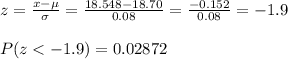
The bolt larger than 18.23 mm is more likely.

5. About 50% of nuts are smaller than 18 mm

6. About 95% of bolts are approximately between 17.804 mm and 18.196 mm

`x_1=\mu-1.96\sigma=18-1.96*0.1=17.804\\\\x_1=\mu+1.96\sigma=18+1.96*0.1=18.196`

7. About 99.7 % of nuts are approximatelly between 17.7 mm and 18.3 mm

`x_1=\mu-3\sigma=18-3*0.1=17.7\\\\x_1=\mu+3\sigma=18+3*0.1=18.3`