Question

Given that exactly two of the six rolls resulted in a 1, find the probability that the first roll resulted in a 1. Note: Your answer should be a number. Do not enter "!" or combinations in your answer.

Answer 1

1/3 or 0.333

Explanation:

If we know that exactly 2 of the 6 rolls resulted in a 1. Then the number of ways to arrange the two 1s into 6 slots is

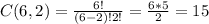`C(6,2) = (6!)/((6-2)!2!) = (6*5)/(2) = 15` ways

Of these 15 ways, some of them have 1 at the 1 slot.

The number of ways to arrange the two 1s so that one 1 is in the 1st slot is 5. Because the 2nd 1 is in any of the other 5 slots.

Therefore, the probability that the first roll resulted in a 1,given that exactly two of the six rolls resulted in a 1 is

5 / 15 = 1/3 or 0.333