Answer:
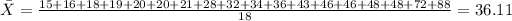

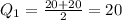
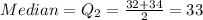
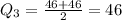
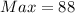
The IQR is
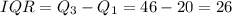
We can find the usual limits for the values and we got:
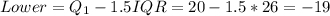
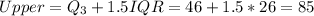
So then the potential outliers for this case is just 88>85
Explanation:
For this case we have the following data:
15 16 18 19 20 20 21 28 32 34 36 43 46 46 48 48 72 88
The sample size is n =18
We can calculate the mean with the following formula:
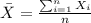
And if we replace we got:
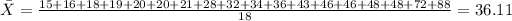
The minimum for this case is

Now we can find the 5 number summary.
For the first quartile we work with the first 10 observations: 15 16 18 19 20 20 21 28 32 34. And Q1 would be the average between the 5th and 6th position of the data ordered, on this case:
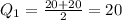
For the median since we have an even number for the sample size would be the average between the 9th and the 10th position from the dataset ordered and we got:
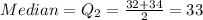
For the third quartile we work with the last 10 observations: 32 34 36 43 46 46 48 48 72 88. And Q3 would be the average between the 5th and 6th position of the data ordered, on this case:
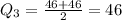
The maximum is
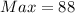
The IQR is
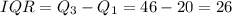
We can find the usual limits for the values and we got:
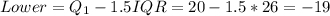
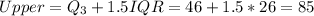
So then the potential outliers for this case is just 88>85