Question

Assume each newborn baby had a probability of approximately 0.54 of being female and 0.46 of being male. For a family of four children, let X = number of children who are girls. Use StatCrunch to find the probability that the family has two girls and two boys. Please note: You must enter your answer as a decimal (not a percentage) rounded to four decimal places with a zero in front of the decimal point. Example: 0.6547

Answer 1

0.3702

Explanation:

For each newborn, there are only two possible outcomes. Either it is a female, or it is a male. The probability of a newborn being male or female is independent from other newborns. So we use the binomial probability distribution to solve this problem.

Binomial probability distribution

The binomial probability is the probability of exactly x successes on n repeated trials, and X can only have two outcomes.

`P(X = x) = C_(n,x).p^(x).(1-p)^(n-x)`

In which
`C_(n,x)` is the number of different combinations of x objects from a set of n elements, given by the following formula.

`C_(n,x) = (n!)/(x!(n-x)!)`

And p is the probability of X happening.

Assume each newborn baby had a probability of approximately 0.54 of being female and 0.46 of being male. For a family of four children, let X = number of children who are girls.

This means that
`p = 0.54, n = 4`

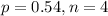
Find the probability that the family has two girls and two boys.

Two girls, so
`P(X = 2)`

`P(X = x) = C_(n,x).p^(x).(1-p)^(n-x)`

`P(X = 2) = C_(4,2).(0.54)^(2).(0.46)^(2) = 0.3702`

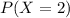
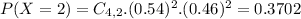
The answer is 0.3702