Question

Triangle ABC is given where MLA = 33°, a = 15 in, and the height, h, is 9 in. How many distinct triangles can be made with the given measurements? Please help :(

Answer 1

One triangle

Explanation:

step 1

Find the measure of side AC

In the right triangle of the left

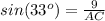`sin(33^o)=(9)/(AC)` ----> by SOH (opposite side divided by the hypotenuse)

`AC=(9)/(sin(33^o))=16.5\ in`

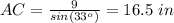
step 2

Find the measure of angle B

Applying the law of sines

`(a)/(Sin(A))=(b)/(Sin(B))`

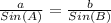
substitute the given values

`(15)/(Sin(33^o))=(16.5)/(Sin(B))`

`Sin(B)=(16.5)/(15)Sin(33^o)=0.60`

`B=sin^(-1)(0.60)= 36.87^o`

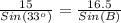
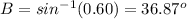
step 3

Find the measure of angle C

Remember that the sum of the interior angles of a triangle must be equal to 180 degrees

so

`33^o+36.87^o+ C=180^o`

`C=110.13^o`

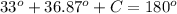
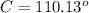
step 4

Find the measure of side AB

Applying the law of sines

`(a)/(Sin(A))=(c)/(Sin(C))`

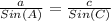
substitute the given values

`(15)/(Sin(33^o))=(c)/(Sin(110.13^o))`

`c=15(Sin(110.13^o))/(Sin(33^o))=25.9\ m`

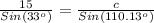
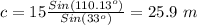
Each measure of the ABC triangle can only have one value, therefore only one triangle can be make with the given measures