Answer:
A) 580 N B) 5,220 J C) 1,740 W D) 870W
Step-by-step explanation:
A)
- Assuming no friction present (or that is negligible) the force exerted by the dogs on the sled must meet Newton's 2nd law:
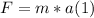
- We can find the value of the acceleration (assumed to be constant), just applying the definition of acceleration, as follows:

- where v = 6.0 m/s, v₀=0, t(0)=0, t=3.0s
- Replacing by the values above, and solving for a:
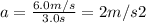
- Replacing this value of a , and m= 290 kg, in (1), we get:

- The magnitude of the force exerted by the dogs on the sled is 580 N.
B)
- In order to be able to find the work done during the 3.0 s, we need to find the displacement produced by the force during that time.
- As the acceleration is assumed to be constant, and the sled starts from rest, we can use the following kinematic equation:

- Replacing by the values of a and t, we can find the displacement x (assuming x₀ = 0), as follows:
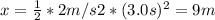
- So, the work done by the force F can be found as follows:
- W = F * d = 580 N* 9 m = 5,220 J
- (An identical outcome could have been found applying the work-energy theorem).
C)
- The instantanous power can be calculated as follows:
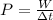
- Replacing W= 5,220 J and t = 3.0 s, we have:
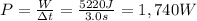
- The instantaneous power, at the end of the 3.0 s, is 1,740 W.
D)
- We can find the instantaneous power at t=1.5 s, using the same equation as above:
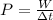
- Now, we need first to know the work done during the first 1.5s.
- We need to find the displacement at the end of the 1.5s time:
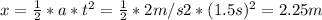
- We can find now the work done at the end of the first 1.5s, as follows:
- W = F * d = 580 N * 2.25 m = 1,305J
- The instantaneous power can be found as follows:
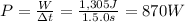
- The instantaneous power, at the end of the first 1.5s, is 870 W.