Answer:
Required Probability = 0.01659
Explanation:
We are given that the laptop replacement times have a mean of 3.3 years and a standard deviation of 0.6 years i.e.;
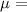 3.3 years and
3.3 years and
 = 0.6 years
= 0.6 years
Sample of 41 is taken i.e. n = 41.
The Z score probability is given by;
Z =
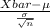 ~ N(0,1)
~ N(0,1)
Let X bar = mean replacement time
So, probability that randomly selected laptop have a mean replacement time of 3.1 years or less = P(X bar <= 3.1 years)
P(X bar <= 3.1) = P(
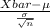 <=
<=
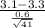 ) = P(Z <= -2.13) = 1 - P(Z < 2.13)
) = P(Z <= -2.13) = 1 - P(Z < 2.13)
= 1 - 0.98341 = 0.01659
Therefore, probability that 41 randomly selected laptops will have a mean replacement time of 3.1 years or less is 0.01659 .