Question

The life span at birth of humans has a mean of 88.37 years and a standard deviation of 18.88 years. Calculate the upper and lower bounds of an interval containing 95% of the sample mean life spans at birth based on samples of 135 people. Give your answers to 2 decimal places.

Answer 1

The correect answers are

85.19, 91.55

Explanation:

The upper bound is given by

μ +z₀.₀₅×
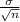`(\sigma)/(√(n) )` = 88.37 +1.96×18.88/√(135) = 91.55

The lower bound is given by

μ -z₀.₀₅×
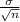`(\sigma)/(√(n) )` = 88.37 -1.96×18.88/√(135) = 85.19

Therefore the upper and lower bound confidence interval containing 95 % of the sample mean are

85.19 , 91.55