Question

A 11.0 µF capacitor is charged to 100 V and is then connected across a 470 Ω resistor only. What is the voltage accross the capacitor after 8.00 ms?

Answer 1

The voltage across the capacitor after 8.00 ms is 35.375 V.

Step-by-step explanation:

Given that,

Capacitor = 11.0 μF

Voltage = 100 V

Resistance = 470 Ω

Time = 8.00 ms

We need to calculate the current

Using formula of current

`I=(q)/(t)`

`I=(CV)/(t)`

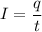
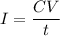
Put the value into the formula

`I=(11.0*10^(-6)*100)/(8.00*10^(-3))`

`I=0.1375\ A`

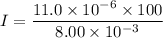
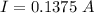
We need to calculate the voltage

Using ohm's law

`V=IR`

`V=0.1375*470`

`V=64.625\ V`

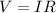
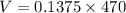
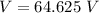
We need to calculate the voltage across the capacitor after 8.00 ms

Using formula of voltage

`V'=100-V`

`V'=100-64.625`

`V'=35.375\ V`

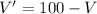
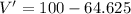
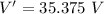
Hence, The voltage across the capacitor after 8.00 ms is 35.375 V.