Question

Tarzan, who weighs 825 N, swings from a cliff at the end of a 19.7 m vine that hangs from a high tree limb and initially makes an angle of 21.5° with the vertical. Assume that an x axis points horizontally away from the cliff edge and a y axis extends upward. Immediately after Tarzan steps off the cliff, the tension in the vine is 768 N. Just then, what are (a) the force from the vine on Tarzan in unit-vector notation, and (b) the net force acting on Tarzan in unit-vector notation? What are (c) the magnitude and (d) the direction (measured counterclockwise from the positive x-axis) of the net force acting on Tarzan? What are (e) the magnitude and (f) the direction of Tarzan's acceleration just then?

Answer 1

a) T = (281.47 i ^ + 714.56 j ^) N , b) F_net = (281.47 i ^ - 110.44 j ^) N ,

c) F = 281.70 N, d) θ = 338.58º , e) a = 3,588 m / s² , f) θ = 201.45º

Step-by-step explanation:

For this exercise we will use Newton's second law on each axis

X axis

-Tₓ = m aₓ

Y Axisy

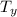`T_(y)` –W = m a_{y}

Let's use trigonometry to find the components of force

sin 21.5 = Tₓ / T

cos 21.5 = T_{y} / T

Tₓ = T sin 21.5

T_{y} = T cos 21.5

Tₓ = 768 sin 21.5 = 281.47 N

T_{y} = 768 cos 21.5 = 714.56 N

a) the force of the rope on Tarzan is

T = (281.47 i ^ + 714.56 j ^) N

b) The net force is the subtraction of the tension minus the weight of Tarzan

Y Axis F_net = 714.56 - 825 = -110.44 N

F_net = (281.47 i ^ - 110.44 j ^) N

c) Let's use Pythagoras' theorem

F = √ (Fₓ² + T_{y}²)

F = √ (281.47² + 110.44²)

F = 281.70 N

d) Let's use trigonometry

tan θ = F_{y} / Fₓ

θ = tan⁻¹ F_{y} / Fₓ

θ = tan⁻¹ (-110.44 / 281.47)

θ = -21.42º

This angle is average clockwise, for counterclockwise measurement

θ = 360 - 21.42

θ = 338.58º

Acceleration

X axis

Tₓ = m aₓ

aₓ = Tₓ / m

The mass of Tarzan is

m = W / g

m = 825 / 9.8 = 84.18 kg

aₓ = 281.47 / 84.18

aₓ = -3.34 m / s2

Y Axis

T_{y}-W = m a_{y}

a_{y} = (T_{y} -W) / m

a_{y} = (714.56-825) / 84.18

a_{y} = - 1,312 m / s²

Acceleration Module

a = √ aₓ² + a_{y}²

a = √ (3.34² +1.312²)

a = 3,588 m / s²

The angle

θ = tan⁻¹ a_{y} / aₓ

θ = tan⁻¹ (-1312 / -3.34)

θ = 21.45º

Notice that the two components of the acceleration are negative, so the angle is in the third quadrant, to measure from the x-axis

θ = 180 + 21.45

θ = 201.45º