Step-by-step explanation:
Formula to calculate refractive index of n is as follows.
n =

where,
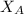 = mole fraction of A
= mole fraction of A
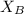 = mole fraction of B
= mole fraction of B
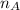 = refractive index of A
= refractive index of A
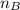 = refractive index of B
= refractive index of B
Hence, putting the given values into the above formula as follows.
n =

1.5248 =
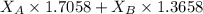 ........ (1)
........ (1)
Also, it is known that
 = 1
= 1
and,
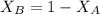 ......... (2)
......... (2)
Now, put equation (2) in equation (1) as follows.
1.5248 =
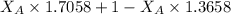
1.5248 =
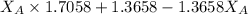
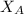 = 0.467
= 0.467
And, the value of
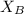 is calculated as follows.
is calculated as follows.
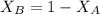
=
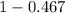
= 0.533
Hence, mole percentage of A will be calculated as follows.
Mole % of A =
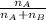
=
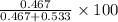
= 46.7%
Thus, we can conclude that the mole percent of A in their mixture is 46.7%.