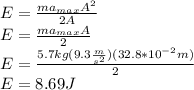Question

A 5.7 kg object oscillates on a spring with an amplitude of 32.8 cm with a maximum acceleration of 9.3 m/s 2 . Find the total energy. Answer in units of J.

Answer 1

`E=8.69J`

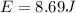
Step-by-step explanation:

The total energy of a mass-spring system is defined as:

`E=(kA^2)/(2)(1)`

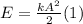
Where k is the spring constant and A is the amplitude of the oscillation. We can calculate k from the natural frequency of this system:

`\omega^2=(k)/(m)\\k=m\omega^2(2)`

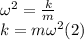
Now, we can calculate
`\omega` from the maximum acceleration:

`a_(max)=A\omega^2\\\omega^2=(a_(max))/(A)(3)`

Replacing (3) in (2):

`k=m(a_(max))/(A)`

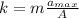
Replacing this in (1):

`E=(ma_(max) A^2)/(2A)\\E=(ma_(max)A)/(2)\\E=(5.7kg(9.3(m)/(s^2))(32.8*10^(-2)m))/(2)\\E=8.69J`