Question

The probability that a boy is born with Down's syndrome is p, 0

Answer 1

The probability that a baby born with Down's syndrome is a boy is
`(p)/(p+q)`.

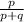Explanation:

The probability of a baby born being a boy (B) or a girl (G) is same, i.e.

P (B) = P (G) = 0.50.

The probability of a boy is born with Down's syndrome is, P (D|B) = p.

The probability of a girl is born with Down's syndrome is, P (D|G) = q.

The law of total probability states that:

`P(X)=P(X|Y)P(Y)+P(X|Z)P(Z)`

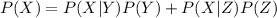
Use this law to compute the probability of a baby born with Down's syndrome as follows:

`P(D)=P(D|B)P(B)+P(D|G)P(G)\\=(p*0.50)+(q*0.50)\\=0.50(p+q)`

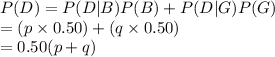
The conditional probability of an event X given that another event Y has already occurred is:

`P(X|Y)=(P(Y|X)P(X))/(P(Y))`

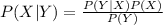
Compute the probability that a baby born with Down's syndrome is a boy as follows:

`P(B|D)=(P(D|B)P(B))/(P(D)) =(p*0.50)/(0.50(p+q)) =(p)/(p+q)`

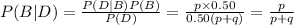
Thus, the probability that a baby born with Down's syndrome is a boy is
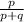`(p)/(p+q)`.