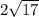The simplest radical form is
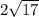
Step-by-step explanation:
The expression is
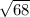
To determine the radical form, let us write the number 68 as a product of prime factors.
Thus, we have,
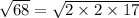
Since, 2, 17 are prime factors and hence, no further factorization is possible.
Hence, it can be written as,
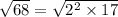
Applying the radical rule
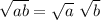 , we have,
, we have,
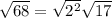
Simplifying, we have,
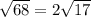
Thus, the simplest radical form is