Answer:
(a) The sample size required is 1034.
(b) The sample size required is 1040.
Explanation:
The confidence interval for population proportion is:

The margin of error is:
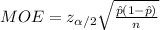
Given:

The critical value of z for 99% confidence level is:
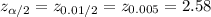 *Use a standard normal table.
*Use a standard normal table.
(a)
Compute the sample size required if the previous estimate is
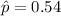 as follows:
as follows:

Thus, the sample size required is 1034.
(b)
Compute the sample size required if there was no previous estimate as follows:
Assume that the estimated proportion be 0.50.
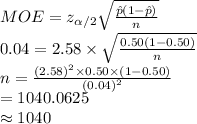
Thus, the sample size required is 1040.