Question

The diagram shows a sketch of the circle C with centre P. The circle has equation (x-2)^2+(y+3)^2=25. Write down the coordinates of P. State the name of the radius of C.

Answer 1

In this case, the coordinates of the center P are (2, -3) and the name of the radius of circle C is 5.

Step-by-step explanation:

The equation of the circle C is given by
`(x-2)^2+(y+3)^2=25.`

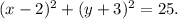
From the equation, we can identify the coordinates of the center of the circle, P. The x-coordinate of the center is the value inside the parentheses after x, which is 2. The y-coordinate of the center is the value inside the parentheses after y, which is -3. Therefore, the coordinates of P are (2, -3).

The radius of the circle is the distance from the center to any point on the circle. In this case, we can see that the equation of the circle is in the form
`(x-h)^2+(y-k)^2=r^2`, where (h,k) represents the coordinates of the center and r represents the radius. Comparing the given equation to this form, we can conclude that the radius of circle C is 5.

To summarize:

- The coordinates of the center P are (2, -3).

- The name of the radius of circle C is 5.

Answer 2

P (2,-3), r = 5

Step-by-step explanation:

The equation of a circle is written as

`(x-a)^2+(y-b)^2=r^2`

where:

a is the x-coordinate of the centre of the circle

b is the y-coordinate of the centre

r is the radius of the circle

In this problem, the equation of the circle is:

`(x-2)^2+(y+3)^2=25`

By comparing the two equations, we find that:

`a=2`

`b=-3`

So, the coordinates of the centre P are

P (2,-3)

Also, we see that

`r^2=25 \rightarrow r=5`

So, the radius of the circle is 5.