Answer:
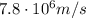
Step-by-step explanation:
When the electron moves, the gain in its kinetic energy is equal to the decrease in its electric potential energy, since the total energy must be conserved.
Therefore, we can write:
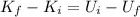
where
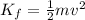 is the final kinetic energy of the electron, where
is the final kinetic energy of the electron, where
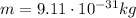 is its mass
is its mass
v is its final speed
 is the initial kinetic energy (the electron starts from rest)
is the initial kinetic energy (the electron starts from rest)
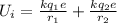 is the initial electric potential energy, where
is the initial electric potential energy, where
k is the Coulomb's constant
 is the charge 1
is the charge 1
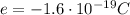 is the electron charge
is the electron charge
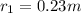 is the initial distance of the electron from charge 1
is the initial distance of the electron from charge 1
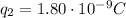 is charge 2
is charge 2
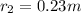 is the initial distance of the electron from charge 2
is the initial distance of the electron from charge 2
 is the final electric potential energy, where
is the final electric potential energy, where
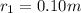 is the final distance between electron and charge 1
is the final distance between electron and charge 1
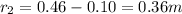 is the final distance between electron and charge 2
is the final distance between electron and charge 2
Substituting everything we find:
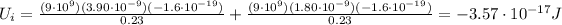
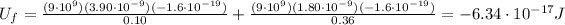
So the final kinetic energy is
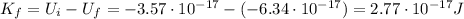
And therefore, the final speed is:
