Answer:
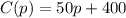
Explanation:
Let p represent number of phones produced in one day.
We have been given that Allison must pay a daily fixed cost to rent the building and equipment, and also pays a cost per phone produced for materials and labor. The daily fixed costs are $400 and and the total cost of producing 3 phones in a day would be $550.
Let x represent cost of each phone.
We can represent this information in an equation by equating total cost of 3 phones with 550 as:
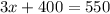
Let us find cost of each phone.
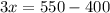
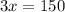
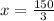

Since cost of each phone is $50, so cost of p phones would be
 .
.
The total cost would be equal to cost of p phones plus fixed cost.
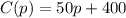
Therefore, our required cost function would be
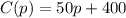 .
.