Question

The heights in inches of males in the united states are believed to be approximately normally distributed with mean u the mean height of a random sample of 25 american adult males is found to be x=69.72 and the standard deviation s=4.15 what is the standard error of x

Answer 1

Standard error = 0.83

Explanation:

Given that the heights in inches of males in the united states are believed to be approximately normally distributed with mean u

Sample mean for 25 adults randomly taken =

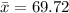`\bar x = 69.72` inches

Std deviation of sample s =
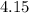`4.15` inches

Since X the height of males is Normal we find that the sample mean also will follow a normal distribution.

By central limit theorem, mean of x would be= sample mean= 69.72

Std error =
`(s)/(√(n) ) =(4.15)/(5) \\=0.83`

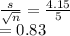
Standard error of the mean = std dev/square root of sample size.

Here only sample std deviation is known

So we used it

Standard error = 0.83