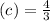Question

A line passes through (2, 2) and is perpendicular to the line 3x + y = 3. Its y- intercept

Answer 1

Explanation:

Given equation of line is:

`3x + y = 3\\\\</p><p>\therefore y = - 3x + 3\\\\`

Equating it with
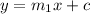`y = m_1x+c` we find:

`m_1=-3`

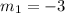
Let the slope of required line be
`m_2`

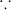`\because` Required line is parallel to the given line.

`\therefore\: m_2=(-1)/(m_1)=(-1)/(-3)=(1)/(3)`

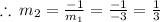
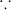`\because` Required line passes through (2, 2) and has a slope
`m_2=(1)/(3)`

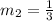
`\therefore` Equation of line in slope point form is given as:

`y - y_1 = m_2(x-x_1) \\\\</p><p>\therefore y- 2=(1)/(3)(x-2)\\\\</p><p> \therefore y- 2=(1)/(3)x-(2)/(3)\\\\</p><p> \therefore y=(1)/(3)x+2-(2)/(3)\\\\</p><p> \therefore y=(1)/(3)x+(2* 3-2)/(3)\\\\</p><p> \therefore y=(1)/(3)x+(4)/(3)\\\\`

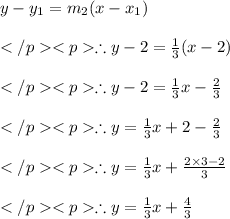
Equating it with
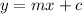`y = mx+c` we find:

y-intercept
`(c) =(4)/(3)`