Answer:
Probability that at most 2 of them are defective = 94.92%
Explanation:
There are 20 lightbulbs and 5 are defective.
Probability of picking a defective ball = 5/20 = 0.25
We need to randomly pick 4 lightbulbs so that at most 2 are defective
We can treat this as a binomial distribution since there are two possibilities: either a defective or a non-defective lightbulb
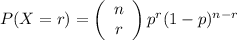
where p = probability of success,
n = number of trials
Since at most 2 lightbulbs are defective, we can either have 0 defective or 1 defective or 2 defective bulbs.
The probability that at most 2 defective lightbulbs from a random draw of 4 lightbulbs is,
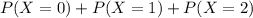
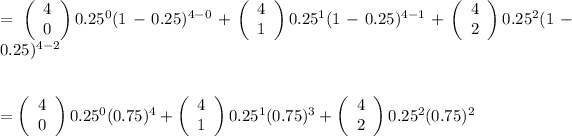
= 0.9492 = 94.92%