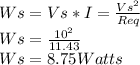Question

Problem 02.061 For the given circuit, assume vS = 10 V, R1 = 9 Ω, R2 = 4 Ω, R3 = 4 Ω, R4 = 5 Ω, and R5 = 4 Ω. Reference Book & Resources Section Break Problem 02.061 5.value: 10.00 points Required information Problem 02.061.b Determine the power delivered by the source vS. (Round the final answer to two decimal places.) The power delivered by the source vS is W.]

Answer 1

Ws=8.75 Watts

Step-by-step explanation:

As per fig. of prob 02.061, it is clear that R5 and R4 are in parallel, its equivalent Resistance will be:

`(1)/(Req45)=(1)/(R4)+(1)/(R5)`

`(1)/(Req45)=(1)/(5)+(1)/(4)`

`(1)/(Req45)=0.2+0.25=0.45\\ Req45=2.22`

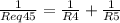
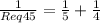
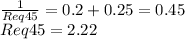
Now, this equivalent Req45 is in series with R3, therefore:

`Req345=R3+Req45\\Req345=4+2.22\\Req345=6.22`

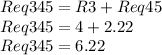
This Req345 is in parallel with R2, i.e

`Req2345=(R2^(-1)+Req345^(-1) )^(-1)\\ Req2345=(4^(-1)+6.22^(-1) )^(-1) \\Req2345=2.43`

Now this gets in series with R1:

`Req12345=R1+Req2345\\Req12345=9+2.43\\Req12345=11.43`

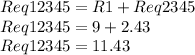
Now, the power delivered Ws is:

`Ws=Vs*I=(Vs^(2))/(Req) \\Ws=(10^(2) )/(11.43) \\Ws=8.75 Watts`