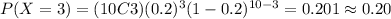Question

In a class of 100 students, 20 students have hard copy and 80 students have electronic textbooks for the course. If you randomly choose 10 students in this class, find the approximate probability using a binomial distribution that 3 of them have hard copy texts (round off to second decimal place).

Answer 1

`P(X=3)`

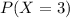
And if we use the probability mass function we got:

`P(X=3)=(10C3)(0.2)^3 (1-0.2)^(10-3)=0.201 \approx 0.20`

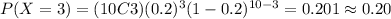
Explanation:

Previous concepts

The binomial distribution is a "DISCRETE probability distribution that summarizes the probability that a value will take one of two independent values under a given set of parameters. The assumptions for the binomial distribution are that there is only one outcome for each trial, each trial has the same probability of success, and each trial is mutually exclusive, or independent of each other".

Solution to the problem

Let X the random variable of interest "number of students with hard copy texts", on this case we now that:

`X \sim Binom(n=10, p=20/100=0.2)`

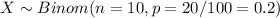
The probability mass function for the Binomial distribution is given as:

`P(X)=(nCx)(p)^x (1-p)^(n-x)`

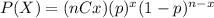
Where (nCx) means combinatory and it's given by this formula:

`nCx=(n!)/((n-x)! x!)`

And we want to find this probability:

`P(X=3)`

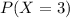
And if we use the probability mass function we got:

`P(X=3)=(10C3)(0.2)^3 (1-0.2)^(10-3)=0.201 \approx 0.20`