Question

8-14 A Cu-30% Zn alloy tensile bar has a strain-hardening coefficient of 0.50. The bar, which has an initial diameter of 1 cm and an initial gage length of 3 cm, fails at an engineering stress of 120 MPa. At the moment of fracture, the gage length is 3.5 cm and the diameter is 0.926 cm. No necking occurred. Calculate the true stress when the true strain is 0.05 cm/cm.

Answer 1

The true stress at true strain 0.05cm/cm is 80MPa

Step-by-step explanation:

Given that

the strength coefficient is K

true strain is ε

strain hardening exponent is n

initial diameter of bar is d = 1cm, (10mm)

tensile force is F

engineering stress(S) = 120

the engineering stress(S) =
`(F)/((\pi )/(4)(d^2) )`

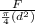
To find force (F) =

120 =
`(F)/((\pi )/(4)(100^(2) ))`

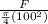
F = 120 * (π/4) * (100)

F = 9425N

Calculate the true strain (ε) = In (l₀ / l₁)

where

l₀ = initial length of the metallic bar = 3cm

l₁ = final length of metallic bar = 3.5cm

ε = In (3.5 / 3)

= In 1.1667

= 0.154cm/cm

Calculate the true stress (σ) at fracture point

=
`(F)/((\pi )/(4)(d^2) )}`

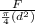
tensile force is F and final diameter of bar is d₁ (d in the eqn)

Substitute 9425 N for F and 0.926 cm (9.26mm) for d₁ (d in the eqn)

σ =
`(9425)/((\pi )/(4)(9.26^2) )}`

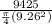
= 140MPa

To find the strength coefficient (K) of the material bar

K =
`(140)/(√(0.154) )`

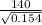
K =
`(140)/(0.3925)`

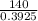
= 356.75MPa

To calculate the true stress σ true strain of 0.05cm/cm

K = 356.75MPa

σ =
`356.75(0.05)^0^.^5`

=
`356.75 ( 0.2236)`

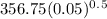
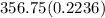
= 80MPa

The true stress at true strain 0.05cm/cm is 80MPa