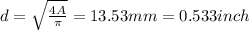Question

A single-phase load is located 2800 ft from its source. The load draws a current of 86 A and operated on 480 V. The maximum voltage drop at the load cannot be greater than 3%. What size aluminum conductors should be installed to operate this load?

Answer 1

Step-by-step explanation:

Initially, we should analyse the system- load and wire are connected in series, which means that they have the same current flow, but we have a voltage drop across the wire.

As it is given, wire is made from aluminum with the specific resistance of 2.82 Ohm*m.

As the specific resistance is given in SI units, we can convert distance in the meters to make calculations easier:

`l=2800 ft=853.44 m`

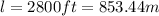
As, the maximum loss of the voltage is 3% and the operating voltage is 480V, we can calculate the maximum allowed drop across the wires:

Vloss=480*3%=14.4 V

Using Ohm's law, we can calculate the maximum allowed resistance of the wire for the given current:

`R=(V_(Loss) )/(I)=14.4/86=0.1674 Ohm`

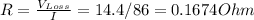
Resistance of the wire, can be calculated using its specific resistance D, length l and area A:

`R=(Dl)/(A)`

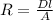
From this equation, we can calculate minimal required area of the wire:

`A=Dl/R=143.769 mm^(2)`

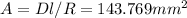
From where, assuming, that the wire has circular cross section, we can find its diameter:

`d=\sqrt{(4A)/(\pi ) }=13.53 mm=0.533 inch`