Question

What’s the x-intercepts, vertex, y-intercept? y=(x+1)2-16

Answer 1

The vertex is (-1, -16)

x intercepts is : (3, 0) or (-5, 0)

y intercept is (0, -15)

Solution:

Given is:

`y = (x+1)^2 - 16`

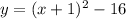
The vertex form of an equation is given as:

`y = a(x-h)^2 + k`

Where, (h, k) is the vertex

On comparing the above equation with given,

h = -1

k = -16

Thus the vertex is (-1, -16)

Find the x intercept:

To find the x-intercept, substitute y = 0 in given

`0 = (x + 1)^2 - 16\\\\x^2 + 2x + 1 - 16 = 0\\\\x^2 + 2x - 15 = 0\\\\Split\ the\ middle\ term\\\\x^2 - 3x +5x - 15 = 0\\\\(x^2 -3x) + (5x - 15) = 0\\\\x(x - 3) + 5(x - 3) = 0\\\\Factor\ out\ x - 3\\\\(x-3)(x + 5) = 0\\\\x = 3\\\\x = -5`

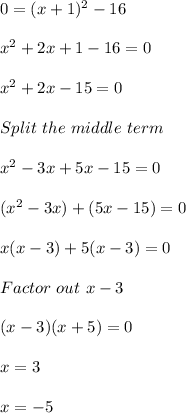
Thus x intercepts is : (3, 0) or (-5, 0)

Find the y intercept:

To find the y-intercept, substitute x = 0 in given

`y = (0+1)^2 - 16\\\\y = 1 - 16\\\\y = -15`

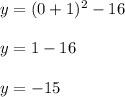
Thus the y intercept is (0, -15)