Question

What is the composition, in atom percent, of an alloy that contains a) 44.5 lbm of silver, b) 84.7 lbm of gold, and c) 7.3 lbm of Cu? The atomic weights for silver, gold, and copper are, respectively, 107.87, 196.97, and 63.55 g/mol.

Answer 1

Answer: The atom percent of silver, gold and copper in the alloy is 43.08 %, 44.93 % and 11.99 % respectively

Step-by-step explanation:

To convert the given masses into grams, we use the conversion factor:

1 lb = 453.6 grams

To calculate the number of moles, we use the equation:

`\text{Number of moles}=\frac{\text{Given mass}}{\text{Molar mass}}` ......(1)

To calculate the atom percent of substance in sample, we use the equation:

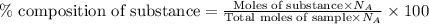`\%\text{ composition of substance}=\frac{\text{Moles of substance}* N_A}{\text{Total moles of sample}* N_A}* 100` ......(2)

where,

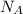`N_A` = Avogadro's number

• Moles of Silver:

Mass of silver = 44.5 lb = 20185.2 grams

We know that:

Molar mass of silver = 107.87 g/mol

Putting values in equation 1, we get:

`\text{Moles of silver}=(20185.2g)/(107.87g/mol)=187.12mol`

• Moles of Gold:

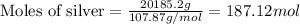
Mass of gold = 84.7 lb = 38419.9 grams

We know that:

Molar mass of gold = 196.97 g/mol

Putting values in equation 1, we get:

`\text{Moles of gold}=(38419.9g)/(196.97g/mol)=195.05mol`

• Moles of Copper:

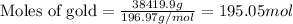
Mass of copper = 7.3 lb = 3311.3 grams

We know that:

Molar mass of copper = 63.55 g/mol

Putting values in equation 1, we get:

`\text{Moles of copper}=(3311.3g)/(63.55g/mol)=52.10mol`

Total moles of the sample =

• For Silver:

Moles of silver = 187.12 moles

Total moles = [187.12 + 195.05 + 52.10] = 434.27 moles

Putting values in equation 2, we get:

`\%\text{ composition of silver}=(187.12* N_A)/(434.27* N_A)* 100\\\\\%\text{ composition of silver}=43.08\%`

• For Gold:

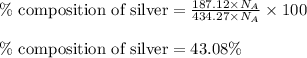
Moles of gold = 195.05 moles

Total moles = [187.12 + 195.05 + 52.10] = 434.27 moles

Putting values in equation 2, we get:

`\%\text{ composition of gold}=(195.05* N_A)/(434.27* N_A)* 100\\\\\%\text{ composition of gold}=44.93\%`

• For Copper:

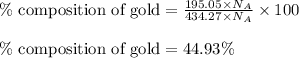
Moles of copper = 52.10 moles

Total moles = [187.12 + 195.05 + 52.10] = 434.27 moles

Putting values in equation 2, we get:

`\%\text{ composition of copper}=(52.10* N_A)/(434.27* N_A)* 100\\\\\%\text{ composition of copper}=11.99\%`

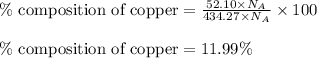
Hence, the atom percent of silver, gold and copper in the alloy is 43.08 %, 44.93 % and 11.99 % respectively