Question

1. What is the area of this polygon?

Enter your answer in the box.
[ ] units²
2. What is the area of this polygon?
A) 28.5 units²
B) 34.5 units²
C) 37.5 units²
D) 40.5 units²
3. The curved part of this figures is a semicircle.
What is the best approximation for the area of this figure?
a) 14+16.25π units²
b) 28+16.25π units²
c) 28+8.125π units²
d) 14+8.125π units²
4. This figure is made up of a rectangle and parallelogram.
What is the area of this figure?
Enter your answer in the box. Do not round any side lengths.
[ ] units²
5. What is the area of this composite shape?
Enter your answer in the box.
[ ] in²

Answer 1

1. 45 units
2. 35.5

3. 14+8.125π units²

4. 26

5. 62

Explanation:

I took the test.

Answer 2

1) 31.5

2) C) 37.5 units²

3) a) 14+16.25π units²

4) 25.5 square units

5) 40 units²

Explanation:

1. The area of the polygon can be found by counting the number of unit squares inside the polygon.

We have 31.5 unit squares. Therefore the area is 31.5 square units.

2. We still count the square units. This time, we have 37.5 unit squares.

Therefore the area is 37.5 units²

The correct answer is C

3. The area of a semi-circle is given by

`\frac{\pi \: {r}^(2) }{2}`

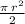
From the diagram, the diameter of the semi- circle is

`d = \sqrt{ {4}^(2) + {7}^(2) }`

`d = 8.06`

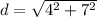
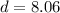
This implies that:

`r = 4.03`

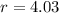
The area of the semi-circle is

`\frac{ {4 .03}^(2) \pi}{2} = 16.25\pi`

The area of the triangular part is ½*7*4=14 square units.

Therefore the area of the figure is:

14+16.25π square units

5. The area of the composite figure is the area of the triangular portion plus the area of the rectangular portion.

The area of the rectangular portion is 5*7=35 square units.

The area of the triangular portion is ½*2*5=5 square units.

The area of the composite figure is 5+35=40 square units.