Question

Bridge is a game played by 4 players with a standard 52 playing card deck of cards. Each

player is dealt 13 cards from the deck and order doesn't matter.

(b) How many different bridge hands are possible if they are required to have all four
aces?
(c) How many different bridge hands are possible if at least one card is an ace?
(d) How many bridge "games" are possible, namely, in how many ways can the 52
cards be distributed to four players?

Show all steps. Please Don't skip any steps. NEED this asap.

Answer 1

Part b: The number of ways in which there will be four aces is 1677106641

Part c: The number of ways in which there will be at least one ace is 442085310304

Part d: The number of ways in which cards can be dealt is 635013559600

Explanation:

Part b

The number of different bridge hands with four aces is

As Total Number of Hands with 4 aces is given as

As the order does not matter, thus the number of Hands with 4 aces is given as

`n_(aces)=^(4)C_(4)+^(48)C_(9)=(4!)/(4!(4-4)!)+(48!)/(9!(48-9)!)\\n_(acesl)=(4!)/(4!(0)!)+(48!)/(9!(39)!)\\\\n_(aces)=1677106641`

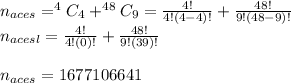
So the number of ways in which there will be four aces is 1677106641

Part c

Total cards without ace = 48

Number of hands of (no ace) =
`n_(no aces)=48C13=192928249296`

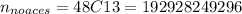
Number of hands of (at least one ace)

`n_(total) - n_(no-aces) \\= 635013559600-192928249296 \\= 442085310304`

So the number of ways in which there will be at least one ace is 442085310304

Part d

As Total Number of Hands is given as

Total Cards=52

Cards per Player=13

As the order does not matter, thus the number of Hands is given as

`n_(total)=^(52)C_(13)=(52!)/(13!(52-13)!)\\n_(total)=^(52)C_(13)=(52!)/(13!(39)!) \\n_(total)=^(52)C_(13)=635013559600\\`

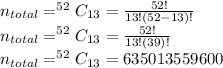
So the number of ways in which cards can be dealt is 635013559600