Question

Right equation of the line that is perpendicular to the line Y equals negative 3X +8 and passes through the point -2, 3. Right equation in point slope form

Answer 1

hello!

=======================================================

Perpendicular lines have slopes that are opposite reciprocals.

This means we take a number, flop it over, and change its sign.

In this case, we take
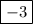`\boxed{-3}`, change its sign:

`\boxed{3}`

3 can also be written as

`\boxed{(3)/(1) }`

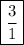
Now the numerator & denominator switch places:

`\boxed{(1)/(3)}`

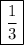
So that's the slope of the new line.

Now, let's write the equation in point-slope form:-

`\bigstar{\underline{\boxed{\pmb{y-y_1=m(x-1)}}}`

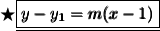
Where

y₁ is the y-coordinate of the point (in this case, y₁ = 3)

m = slope (1/3)

x₁= the x-coordinate of the point (in this case, it's -2)

Plug in the values:-

`\bigstar{\boxed{\pmb{y-3=(1)/(3)(x-(-2)}}`

`\bigstar{\boxed{\pmb{y-3=(1)/(3)(x+2)} }\longleftarrow\sf{Point-Slope~Formula:)}}`

===============================================

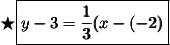
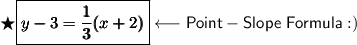
note:-

Hope everything is clear; if you need any clarification/explanation, kindly let me know, and I will comment and/or edit my answer :)