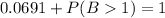Answer:
a) 0.2182
b) 0.0691
c) 0.9309
Explanation:
Binomial probability distribution
The binomial probability is the probability of exactly b successes on n repeated trials, and B can only have two outcomes.
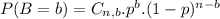
In which
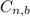 is the number of different combinations of b objects from a set of n elements, given by the following formula.
is the number of different combinations of b objects from a set of n elements, given by the following formula.
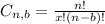
And p is the probability of B happening.
In this problem we have that:
Bin(20,0.2).
This means that
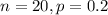
(a) P(B=4).
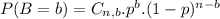
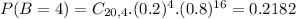
(b) P(B≤1).
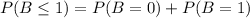
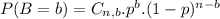

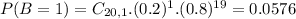
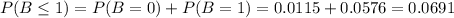
(c) P(B>1).
Either B is less than or equal to 1, or B is larger than 1. The sum of the probabilities of these events is decimal 1. So
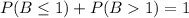
We have that, from b),
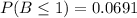
So