Answer:
14.139m/s
Step-by-step explanation:
For a body moving along a curve on a surface whose coefficient of friction is
 , the maximum velocity v the body can sustain beyond which it would skid off is given by equation (1);
, the maximum velocity v the body can sustain beyond which it would skid off is given by equation (1);
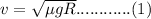
where g is acceleration due to gravity taken as
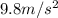 and R is the radius of the curve.
and R is the radius of the curve.
Given;
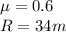
Hence'
