Question

You can insure a $42,000 diamond for its total value by paying a premium of D dollars. If the probability of loss in a given year is estimated to be 0.02, what premium should the insurance company charge if it wants the expected gain to equal $1,000?

Answer 1

`E(X) =\sum_(i=1)^n X_i P(X_i)`

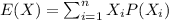
Replacing the values that we have:

`1 = 0.98*a + 0.02(a-42) = 0.98a +0.02a -0.84`

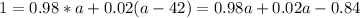
And solving for a we got:

`1.84 = a`

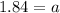
So then the premium value for the insurance on this case should be 1840 dollars.

Step-by-step explanation:

For this case we can define the random variable X as the gain ( in thousand of dollars) of insurance company

We assume that the premium clase charge and amount of a to the company and we know from the info given that:

`p(X=a) = 1-0.02 = 0.98`

`p(X = a-42) = 0.02`

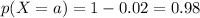
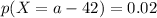
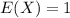`E(X) = 1` represent the expected gain in thousand of dollars

The expected value of a random variable X is the n-th moment about zero of a probability density function f(x) if X is continuous, or the weighted average for a discrete probability distribution, if X is discrete.

And using the definition for a discrete random variable we know that :

`E(X) =\sum_(i=1)^n X_i P(X_i)`

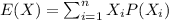
Replacing the values that we have:

`1 = 0.98*a + 0.02(a-42) = 0.98a +0.02a -0.84`

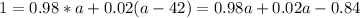
And solving for a we got:

`1.84 = a`

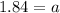
So then the premium value for the insurance on this case should be 1840 dollars.