Question

A city's water supply is contaminated with a toxin at a concentration of 0.63 mg/L. Fortunately, this toxin decomposes to a safe mixture of products by first-order kinetics with a rate constant of 0.27 day–1. How long will it take for half of the toxin to decompose?

Answer 1

t = 2,57 days

Step-by-step explanation:

A reaction follows the first-order kinetics when attend the formula:

`ln([A]_t)/([A]_0) = -kt`

Where [A]₀ is the initial concentration of the toxin (0,63mg/L) and [A]t is the half of the initial concentration (0,315mg/L). k is 0,27 day⁻¹ and t is the time the descomposition takes. Replacing:

`ln(0,315)/(0,63) = -0,27day^(-1)t`

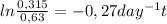
t = 2,57 days

I hope it helps!