Question

If the sides of a square are increasing by 3 in., the area becomes 64 in square. Find the length of a side of the original square

Answer 1

Original side of the square =5 inches

Explanation:

The side of the square is increasing by 3

Let the original side of the square be 'a' inches.

GIVEN:

On increasing side by 3 side becomes=a+3 inches

On increasing the side the area becomes 64 square inches

Area of a square=
`side*side`

`64= (a+3)(a+3)\\64=a^2+3a+3a+9\\64=a^2+6a+9\\a^2+6a=64-9\\a^2+6a=55\\a^2+6a-55=0\\a^2-5a+11a-55=0\\a(a-5)+11(a-5)=0\\(a-5)(a+11)=0\\a=5 \\or\\a=-11`

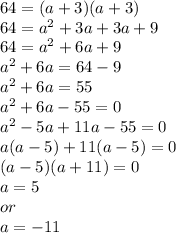
As the side cannot be negative, so the original side of the square is 5 inches