Answer:
The atomic radius of sodium is 185.59 pm.
Sodium metal will float in water.
Step-by-step explanation:
a) To calculate the density of metal, we use the equation:
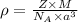
where,
 = density =
= density =
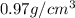
Z = number of atom in unit cell = 2 (BCC)
M = atomic mass of metal = 22.99 g/mol
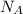 = Avogadro's number =
= Avogadro's number =
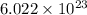
a = edge length of unit cell = ?
Putting values in above equation, we get:
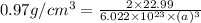
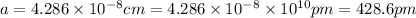
To calculate the radius, we use the relation between the radius and edge length for BCC lattice:
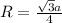
where,
R = radius of the lattice = ?
a = edge length = 428.6 pm
Putting values in above equation, we get:
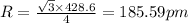
The atomic radius of sodium is 185.59 pm.
b)
Density of water = D = 1 g/mL =
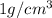
(
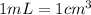 )
)
Density of the sodium metal = d =
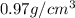
D > d ( float)
Since, the density of the sodium metal is less than the water's density which means that sodium metal will float in water.