Question

Help please

Find the equation of a line that joins the midpoint of (7, 3) and (9, -7) and the y-intercept of
5x – 6 = 2y.​

Answer 1

y =
`(1)/(8)` x - 3

Explanation:

Use the midpoint formula

Given (x₁, y₁ ) and (x₂, y₂ ) then the midpoint is

[
`(1)/(2)` (x₁ + x₂ ),
`(1)/(2)` (y₁ + y₂ ) ]

Here (x₁, y₁ ) = (7, 3) and (x₂, y₂ ) = (9, - 7), thus

midpoint = [
`(1)/(2)` (7 + 9),
`(1)/(2)` (3 - 7 ) ] = (8, - 2)

-----------------------------------------------------------

The equation of a line in slope- intercept form is

y = mx + c ( m is the slope and c the y- intercept )

Rearrange 5x - 6 = 2y into this form by dividing all terms by 2

y =
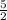`(5)/(2)` x - 3 ← in slope- intercept form

with y- intercept c = - 3 ⇒ (0, - 3 )

-------------------------------------------------------------

Calculate m using the slope formula

m = (y₂ - y₁ ) / (x₂, x₁ )

with (x₁, y₁ ) = (8, - 2) and (x₂, y₂ ) = (0, - 3)

m =
`(-3+2)/(0-8)` =
`(-1)/(-8)` =
`(1)/(8)`

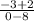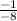y =
`(1)/(8)` x - 3 ← equation of line