Question

An engineer has designed a valve that will regulate water pressure on an automobile engine. The valve was tested on 170 engines and the mean pressure was 7.5 pounds/square inch (psi). Assume the population variance is 0.36. The engineer designed the valve such that it would produce a mean pressure of 7.4 psi. It is believed that the valve does not perform to the specifications. A level of significance of 0.02 will be used. Find the value of the test statistic. Round your answer to two decimal places.

Answer 1

2.17

Explanation:

As the population variance is known we can calculate population standard deviation and so, z-test statistic will be used.

`z=(xbar-pop mean)/((pop SD)/(√(n) ) )`

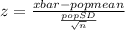
population variance=σ²=0.36

population standard deviation=σ=√0.36=0.6

The value of population mean can be achieved through null hypothesis.

Null hypothesis : μ=7.4

Alternative hypothesis : μ≠7.4

population mean=μ=7.4

xbar=7.5

n=170

z=(7.5-7.4)/(0.6/√170)

z=0.1/(0.6/13.038)

z=0.1/0.046

z=2.17

Thus, the calculated z-test statistic is 2.17.