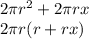Answer:
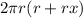
Step-by-step explanation:
Let the area of one circular side be given by the formula :
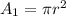
However, the wire is a solid cylinder, then it means that the total area is 2 ×
 =
=

However, there is the surface area to consider. This is the curved area of the wire. This is given as:
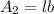
The length is x.
The breadth is calculated as follows - the length of the circle =

Then the area = lb
=
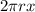
Therefore, the total area is given as
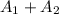
=