Question

Nationwide survey of college seniors by the University of Michigan revealed that almost 70% disapprove of daily pot smoking, according to a report in Parade.If 12 seniors are selected at random and asked their opinion, find the probability that the number who disapprove of smoking pot daily is

anywhere from 7 to 9;

at most 5;

not less than 8.

Answer 1

The probability of number who disapprove of smoking pot daily is from 7 to 9 is 0.6293.

The probability of number who disapprove of smoking pot daily is at most 5 is 0.038601

The probability of number who disapprove of smoking pot daily is is not less than 8 is 0.723655.

Explanation:

The number of seniors that disapprove smoking pot daily X follows binomial distribution with n=12 and p=0.7 because

1. The opinion of seniors are independent.

2. There are two possible outcome approve or disapprove.

3. The probability of seniors disapproves smoking pot daily is constant for each trail i.e. p=0.7.

4. The opinion is asked from 12 senior i.e. fixed number of trails.

The probability of the number of seniors that disapprove smoking pot daily can be computed as

`P(X=x)=nCxp^(x) q^(n-x)`

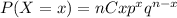
Here, n=12, p=0.7 and q=0.3.

P(number who disapprove of smoking pot daily is from 7 to 9)=P(7≤X≤9)=?

P(7≤X≤9)=P(X=7)+P(X=8)+P(X=9)

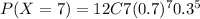`P(X=7)=12C7(0.7)^(7) 0.3^(5)` = 0.158496

`P(X=8)=12C8(0.7)^(8) 0.3^(4)` = 0.231140

`P(X=9)=12C9(0.7)^(9) 0.3^(3)` = 0.239700

P(7≤X≤9)=0.158496+0.231140+0.239700

P(7≤X≤9)=0.6293

The probability of number who disapprove of smoking pot daily is from 7 to 9 is 0.6293.

P(number who disapprove of smoking pot daily is at most 5)=P(X≤5)=?

P(X≤5)=P(X=0)+P(X=1)+P(X=2)+P(X=3)+P(X=4)+P(X=5)

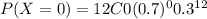`P(X=0)=12C0(0.7)^(0) 0.3^(12)` = 0.000001

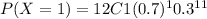`P(X=1)=12C1(0.7)^(1) 0.3^(11)`= 0.000015

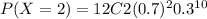`P(X=2)=12C2(0.7)^(2) 0.3^(10)` = 0.000191

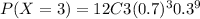`P(X=3)=12C3(0.7)^(3) 0.3^(9)`= 0.001485

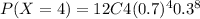`P(X=4)=12C4(0.7)^(4) 0.3^(8)` = 0.007798

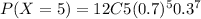`P(X=5)=12C5(0.7)^(5) 0.3^(7)` = 0.029111

P(X≤5)=0.000001+ 0.000015+ 0.000191+ 0.001485+ 0.007798+ 0.029111

P(X≤5)=0.038601

The probability of number who disapprove of smoking pot daily is at most 5 is 0.038601.

P(number who disapprove of smoking pot daily is not less than 8)=P(X≥8)=?

P(X≥8)=1- P(X<8)

P(X≥8)= 1-P(X≤7)

P(X≤7)=P(X≤5)+P(X=6)+P(X=7)

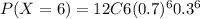`P(X=6)=12C6(0.7)^(6) 0.3^(6)` = 0.079248

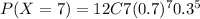`P(X=7)=12C7(0.7)^(7) 0.3^(5)` = 0.158496

P(X≤7)=0.038601+ 0.079248+ 0.158496

P(X≤7)=0.276345

P(X≥8)=1-0.276345

P(X≥8)=0.723655

The probability of number who disapprove of smoking pot daily is is not less than 8 is 0.723655.